1. To explain basic concepts of backgammon. A lot of this will be familiar to many readers. But we're not going to assume anything.

2. To give readers the tools to make use of other backgammon-related material. Mathematically-inclined players will find tremendous value in understanding how match equity tables can improve your doubling decisions. But unless someone stops long enough to explain what a "match equity table" is, it won't be of much value.

3. To introduce the concept of "reference positions." By this, we mean two things. Some are common positions that arise often, and players should know how to handle. The second type of reference position is one that is on the borderline. Perhaps the borderline between a double and no double, between a take and a drop, between playing safely for a win and aggressively for a gammon.

In this article, we're going to focus on #1, and go over some basic probabilities, for the dice and the doubling cube.

Dice Probabilities

Let me start with a rough copy of the page that was my first backgammon lesson:

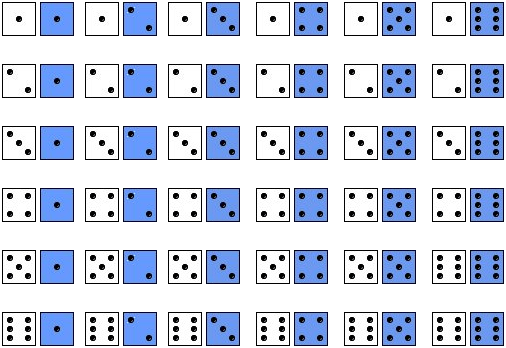
To the backgammon expert, this is—well, less than trivial. But it's surprising how many beginning and intermediate players do not undrestand this display. Pretty much every player understands that it's much easier to roll a number of 6 or lower than one 7 or higher. But for many it ends there. I've had several players who think that the odds on a 6-2 or a 6-6 are both 1 in 21, because there are 21 different ways two dice can come up.

This display doesn't really require a lot of explanation. But let's look at a few of the implications.

Throughout this article we will refer to the number of rolls as the number of ways a particular number or numbers can come up on two dice. So, for example, if we refer to the fact that an 8 can be made by "6 rolls" we mean—that it can come up as a result of 6-2 (2 rolls), 5-3 (2 rolls), 4-4 (1 roll), or 2-2 (1 roll).

First, let's look at the number of ways a particular number can come up on two dice:

1	2	3	4	5	6	7	8	9	10	11	12	15	16	20	24
11	12	14	15	15	17	6	6	5	3	2	3	1	1	1	1

Do you need to memorize this table? Well, sure, it helps, but not really. The important thing to understand is that 6 comes up most often, followed by 5, 4, 3, 2, 1, and then 8, 9, 10, etc. Just understanding that much will help you position yourself better in situations where you need to minimize the number of shots you leave, or maximize your chances of covering a blot.

Time out. Yes, 90% of the readers of this article will know what I mean by the terms shot and blot. But one of the principles of this series is to make sure that all our readers understand all the terminology. Hopefully many will want to read and understand the other articles in this magazine, or other backgammon resources. So even though this article is focused on basic concepts, let's take a moment to clarify a couple terms:

Blot	A checker alone on a pip, which could be sent to the bar if hit by the opponent.
Slot	Slot refers to the act of placing a single checker on a pip, with the intention of placing a second checker there ("covering") in the near future.

Let's also look at the number of rolls that result in at least one of two numbers:

1, 2 20 1, 3 20 1, 4 21 1, 5 22 1, 6 24

2, 3 21 2, 4 23 2, 5 23 2, 6 24 3, 4 24

3, 5 25 3, 6 28 4, 5 26 4, 6 27 5, 6 28

Again, do you need to memorize this? No. But look at the implications. Suppose you have to leave a double shot, and you can leave shots either 2 and 3 pips away, or 6 and 3 pips. If you leave the 2-3 shot, there are 15 rolls that miss. If you leave the 6-3 shot, only 8 numbers miss. You almost double your chances of not being hit, with this one little insight.

The important thing is not to memorize these tables. It is important, though, to understand why there are 36 different combinations, and to be able to make use of this information.

Cube Probabilities

Not that long ago I was playing a match online and a player criticized me. "You give up too easily" he said. "I never like to drop a double when I still have a chance to win."

I don't know what his threshold for "a chance" is, but I know he is giving away a lot of points to his opponents. A surprising number of players don't even know the basic equation for taking or dropping a double.

A very simple rule that will stand you in good stead is this:

You should drop a double when you have less than a 25% chance to win the game. If you have significant chances of being gammoned you should increase the percentage of games you will win; if you will win more than your share of gammons when you do recover to win the game, you can take more freely.

This is really a pretty simple concept. Let's take a very simple position:

1 6

White money game Blue

If you refer back to the dice table above, you will see that Blue has 27 rolls that will get his last checker off, and 9 that don't. He is exactly 25% to win this game.

If he doubles, and you pass, you will lose one point. If you take, then 25% of the time you will win the game, winning two points. Your average gain is a half point (25% times two points). You will lose two points 75% of the time, for an average loss of a point and a half—net, loss of one point. You break even. So this position is right on the border. You can take or pass, and your long-run expectation is about the same.

Let's briefly talk about another one of our concepts—that of "reference positions." Now that you know this position is right on the border, suppose you see this:

1 6

White money game Blue

You could handle this three ways:

1. Be an expert. Know this automatically by heart.

2. Say to yourself "I know I need 25%. Let's see. Anytime he rolls a 5 or 6 that will get both checkers off, so that's 20 rolls. Also, 2-2, 3-3, and 4-4 are good, that's 3 more rolls, for 23. Have I forgotten anything? I hope not. I guess I should take."

3. Think this way: "I know that a position where my opponent needs any six pips is on the border. This can't possibly be worse for me, and it should be better since there are probably some rolls that get a total of six pips and don't get both checkers off. If I could take that one, it has to be right to take this one."

I suggest #3. Partly because it's a lot easier on the brain, partly because you'll get it right more often, and partly because the reason the expert knows this is a take is because that's how HE does it. He knows the 6-pip "reference position" and works from there. See - thinking like an expert isn't all that hard.

So, when should you double? That's actually a fairly complicated question, and it involves some theory we'll discuss in later articles. In general, though:

You should double when you have a solid advantage, and are getting close the to point where you are 75% likely to win.

Many players are horribly afraid of doubling too soon. They worry that their opponent might take, and they'll lose the game, and why lose 2 points when they could have lost just one? Why not wait until they have a huge advantage and cash the game (offer a double the opponent would be foolish to take)?

Well, suppose you have bet on a football game. The teams are evenly matched, there is no point spread, and your team is leading by 3 points going into the final quarter. Would you double the bet if you could? Sure, why not? You have an advantage. You don't need to be up by 14 points with 3 minutes to go, do you?

This doesn't mean you should double with any advantage, however slight. It's a significant disadvantage in a game to have your opponent be able to double, but you can't. But you also don't need to be 90% to win. If you wait for those huge advantages, you will be giving your opponents a lot of chances to win games that they don't deserve.

Suppose you are (only) 80% likely to win the game, and you double. If your opponent drops, you win a point—you've just locked in the other 20% of a game. If he takes, now you will win 2 points 80% of the time, for a net gain of 1.6 points, and lose 2 points 20% of the time, a net loss of 0.4 points, for an average win of 1.2 points. Either way, you're better off.

One excellent rule for doubling is called Woolsey's Law:

If you, put in your opponent's position, would even think of dropping, it must be a good double.

Consider the things that can happen if you double:

1. You have a strong double that your opponent should drop, but he takes. You have just doubled your expected gain on the game. Pretty good return on just turning a cube.

2. You have a double that your opponent should take, but he drops. That's about as good as #1. Since he should take with 25% chances to win, you've just stolen half a point.

3. You have a strong double that your opponent should drop, and he does. Well, that's the idea isn't it? Why let him play on and get lucky?

4. You have a position you shouldn't double in, and he takes. Unless your double was a serious error though, you have doubled the value of the game in a position where you are a solid favorite? How bad can that be?

Advanced readers will very quickly notice that we've oversimplified a number of important cube concepts. We'll deal with them next issue.
 

Next Article: Doubling Theory and Market Losers.

Practical Backgammon is a column for beginning and intermediate players. Its goal is to offer specific solutions to common backgammon situations, and to provide the tools for advancing players to make use of more advanced material.