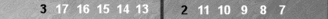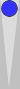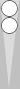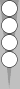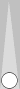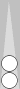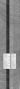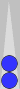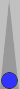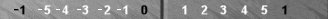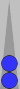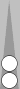|
This is Part 2 of the Naccel 2 series. It is in reponse to Ian's post, though I am writing for the general readership as well.
|
I experimented with Naccel a few years ago, when I first became aware of it, but I found I was returning to Cluster Counting. I can count using this method fairly reliably, but I'm not lightning fast. So I'd love a way to improve.
|
Thanks, Ian, for your comments and position.
Traditional
pip-counting is analogous to walking. Cluster counting is analogous to
running. The beauty of Cluster is that it builds on movements you
already know and enhances them.
Naccel is analogous to skating. It's faster than walking or running, but not
at the beginning. You have to learn how to (a) use the skates properly
first and (b) practice. (Neither is much good without the other.) That
can be tough if nobody is around to show you how to balance, etc., and
in addition you have never seen anyone skate: you don't even know that skating really is faster. It is only natural to lose confidence and go back to running.
Later
in this post, you'll understand enough so that you can watch me skate,
and that might interest you enough to try skating yourself (as you have
never really skated to begin with).
Below is the position you sent.
Let's start with Blue's position. There are dozens of ways to count it
in Naccel, but I'll show you the one that first jumped out at me. You
don't yet know what I know, so I'll build the explanation gradually.
First, review the Supers (Super-points) from Part 1. Now note that in your position here (above), Blue's
back checkers sit on S3 (Super 3) and S2 (Super 2). That's a count of 3
+ 2 = 5 on the far side.
Next, here's a valuable tip: the way to count a stack of six checkers on a point is simply by the point number. For example,
This is a stack of six checkers. (Notice the number "6" on the uppermost checker.)
The trad 6pt is the 0pt in Naccel (marked "0" above). To its right is the 1pt, then the 2pt, and then the 3pt, located at the white point number "3."
Six checkers on the (Naccel) 3pt count 3. That's all there is to it.
Okay, now consider the formation below:
This is one of many ways that six checkers cluster symmetrically around
a point: in this case, the (Naccel) 3pt. The count is "3," just as it
is in the previous diagram. (If that isn't immediately obvious to you,
mentally push the checkers equally towards each other until they're all
stacked on the 3pt in the previous diagram.)
Okay,
now go back and look at your original full-board position. It is made
up almost entirely of two components: (a) the 3 + 2 = 5 count on the
far side, and (b) the 3 count on the near side (just shown). As the 0pt
(trad 6pt) checkers are invisible (they count zero), all that remains
are two checkers on the 1pt, which are 2 pips.
In short, Blue's count is 5 + 3 = 8, with 2 pips left over. I typically write this as "8(2)."
For
reference, the two main subparts of your Blue count are shown below,
with the 2 extra pips in the third diagram. (Subcounts are shown in the
lower right.) Then we'll move on to White's count.
Okay, now let's count White. The position, again, is:
As before, there are many ways of counting. The way that I recommend to
you (for now) is to bring both White's midpoint checkers down 4 pips,
and to compensate, back up her back checkers a combined 8 pips, like
this:
White's near side is a giant poof, similar to the formations in Part 1. It becomes invisible—it counts nothing.
All that remain are White's three back checkers. The one on the roof is
just 1 pip away from S3; she therefore has three on S3 = 9, plus a pip.
Her count is 9(1).
With
Blue's pipcount being 8(2) and White's being 9(1), Blue leads by 5
pips (one shy of a super-pip or supe). That should tell you all you
need to know.
If you want trad totals for some reason, 8 × 6 + 2 + 90 = 140, and 9 × 6 + 1 + 90 = 145.
This
series of posts will continue as long as reader enthusiasm continues
(and assuming I can continue to make the time). Someone can send
another position to count, if/when s/he likes.
Go on to Part 3.
| 


![]()